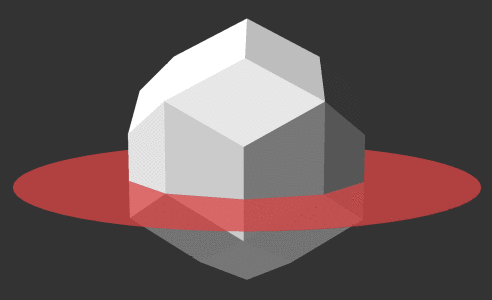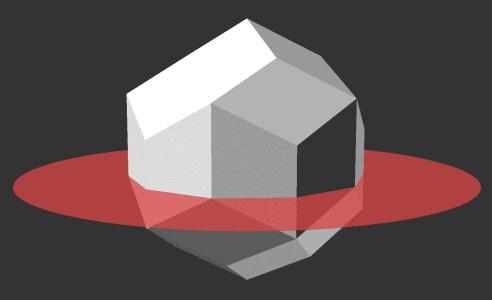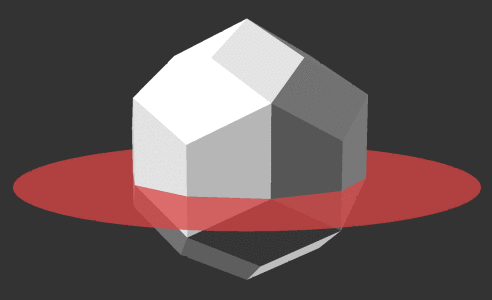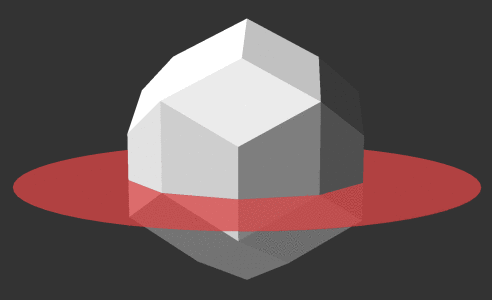

The rhombiyurt is based on the rhombic triacontahedron (RT), a sphere-like polyhedron made of identical diamond-shaped faces. The RT can be "peeled" like an orange into a continuous strip of panels that folds neatly into a compact package. To design a rhombiyurt: (1) slice the RT below the equator, (2) choose a peeling of the upper half that offers a convenient unfurling motion, (3) add hinges along the strip to enable an accordion-like fold.
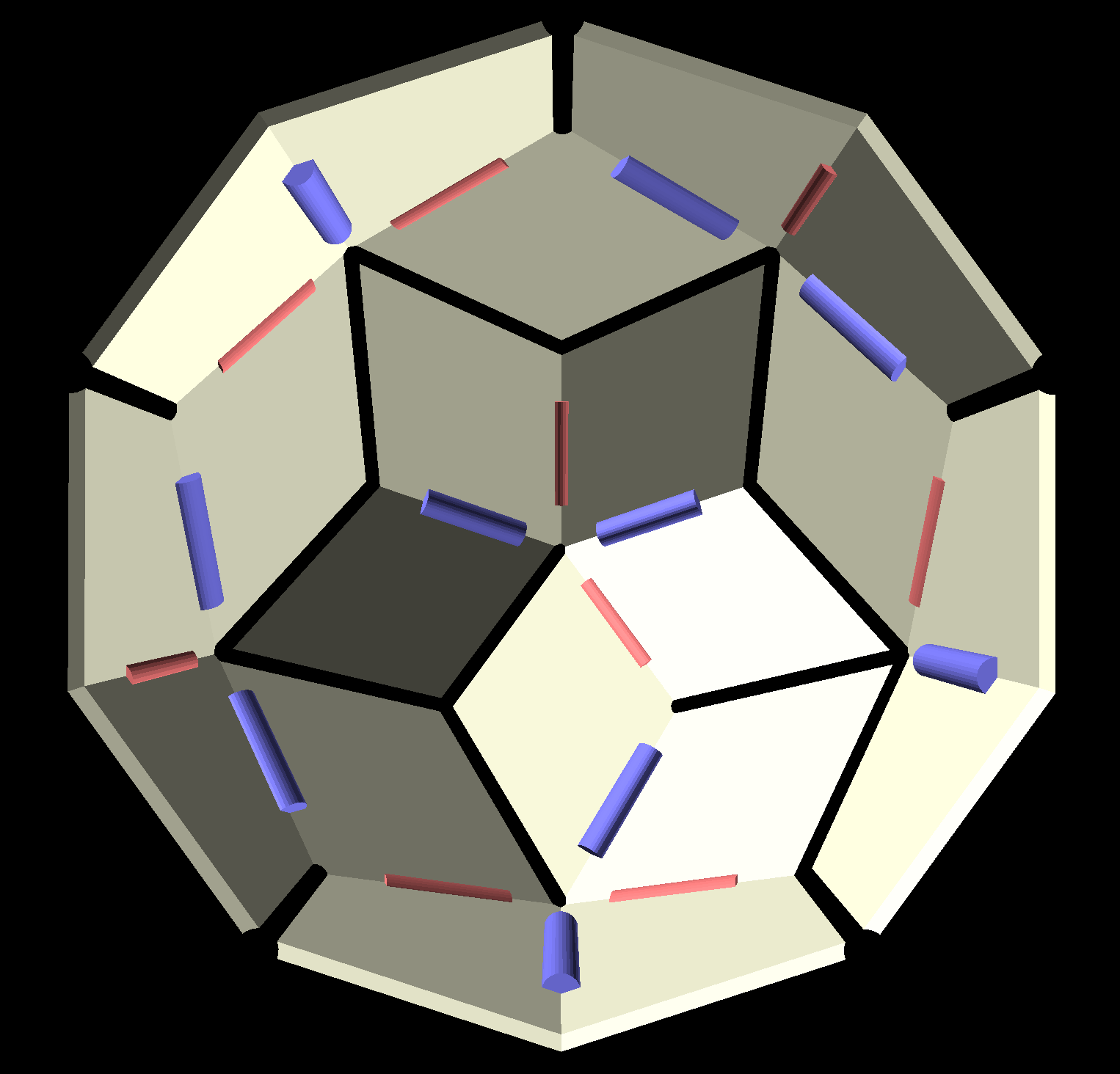
The RT isn't the only "strip-foldable" shape -- see discussion of theory and variations below.
Two 13' (4 meter) diameter rhombiyurts have been our shelter of choice at Burning Man for most of the last decade. With a little practice they can be set up or taken down by one person in <10 minutes.

A rhombiyurt requires significantly less material than a full-sized hexayurt, but provides a more pleasant room-like interior with ample headroom everywhere, better thermal regulation, and superior high-wind stability (due to roundness). Plus, it looks good.
Here is a one-page construction guide (PDF) and build notes. Some pictures from the build:












Please note: Rhombiyurt construction requires more care and planning than a simple hexayurt build. Precise cuts and a well thought out work plan will make a big difference. Measure twice and practice each operation on scrap before doing the real thing.
The rhombic triacontahedron is not the only strip-foldable polyhedron, but it occupies a sweet spot for personal shelters because the panel size is large enough to accommodate (or be) a door, yet small enough that the folded package can be moved around by one person. All Platonic and non-pentagonal Catalan solids can be made to work, notably the disdyakis triacontahedron and disdyakis dodecahedron which offer a zipperable seam and would scale well to larger domes.
More generally: A polyhedron is peelable iff its dual graph has a Hamiltonian path; the peeled strip is neatly foldable iff the polyhedron has reflective symmetry over all hinged edges. Any polyhedron can be made peelable with face cuts (Proof: Add edges to triangulate any non-convex faces, then add an edge from the center of each edge to the center of each face. This yields a degree-4 dual graph, which always has a Hamiltonian path). A slicing of a peelable polyhedron (e.g. to make a dome) will remain peelable if the sliced faces form one or more edge-connected rings. (Proof: The Hamiltonian path is broken into segments by the slicing but since it is non self-crossing, segment ends can be paired and linked around each ring to restore Hamiltonicity.) Some polyhedra don't have edge-peelings that can be laid flat, but any peeling can be folded into a stack whose floor projection (think noon shadow) is no bigger than the polyhedron's biggest face (by introducing hinges into faces as needed). A seam is zipperable if it has no branchings. A conjecture: Every convex polyhedron has a zipperable subdivision peeling that requires no further hinging to fold into a stack with a compact floor projection.
Design by Zintaglio Arts. See some of our past and future BM projects: HeadSpace, Bottle Genii, Mutatis Mutandis, Virascope Alpha, Gnomon and Virascope, FOXP